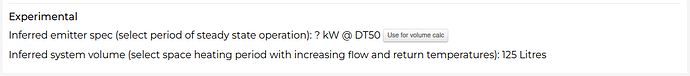
I’ve added an experimental feature to the MyHeatpump app to try and infer radiator spec and system volume.
This can be found at the bottom of the power view, DETAIL section:
How to use it
Indoor temperature sensor & feed required: These calculations require indoor temperature data to work.
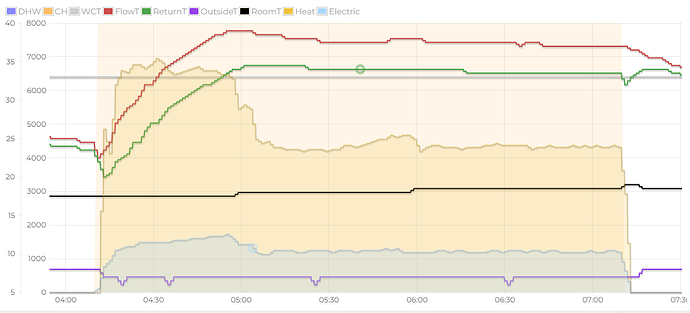
- Select a period with continuous stable output, flow and return temps need to be stable and not increasing during this period e.g 17:20 to 17:30 in the example below:
selected section:
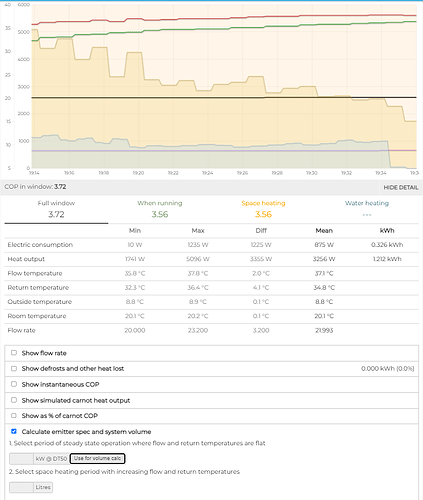
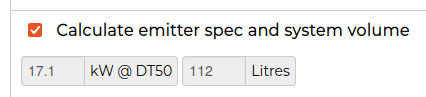
Emitter spec result: 15.6 kW @ DT50 (My heat loss calculation suggests it should be 15.0 kW, so not bad! considering there’s probably a few hundred watts emitted from pipes etc). Not expecting super accuracy here, this is very much ballpark figure. The accuracy is impaired by needing to know an accurate picture of average indoor temperature, a single sensor in the living room is not necessarily representative of the whole house!
DT50 here refers to the standard radiator datasheet output at 70C mean water temperature and 20C room temperature, a difference in temperature of 50K. I know that does not relate to heat pump temperatures but it’s the input required for the radiator output equation https://docs.openenergymonitor.org/heatpumps/basics.html#radiator-output.
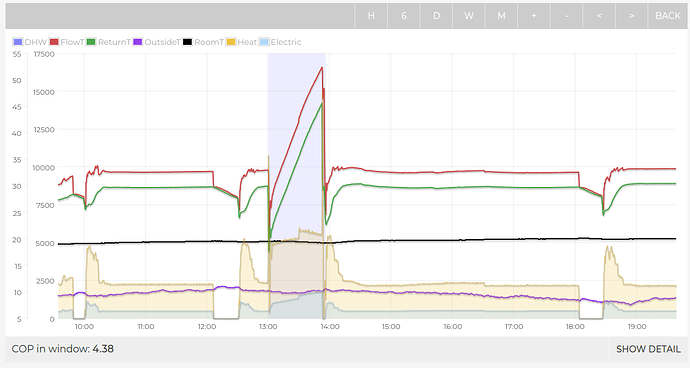
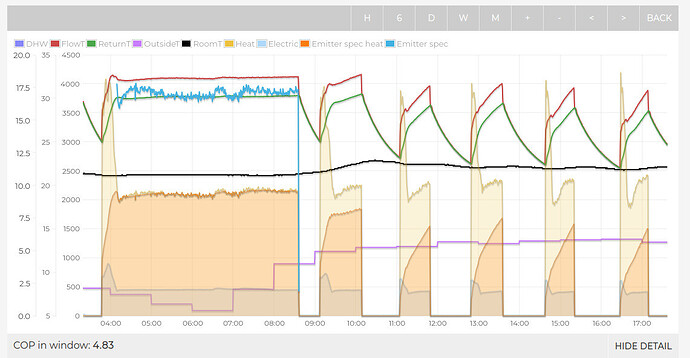
- Click on ‘Use for volume calc’ to save this emitter output value. Zoom out and find a heat pump cycle where the system is warming up (a portion of the heat is going to heating the system volume):
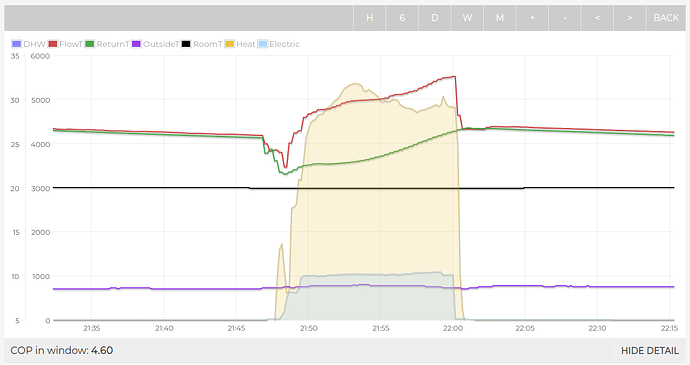
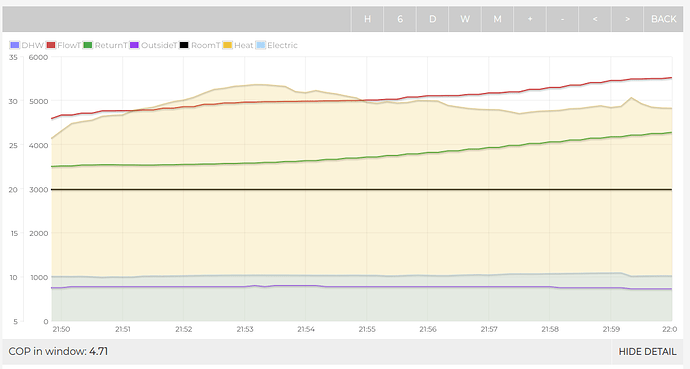
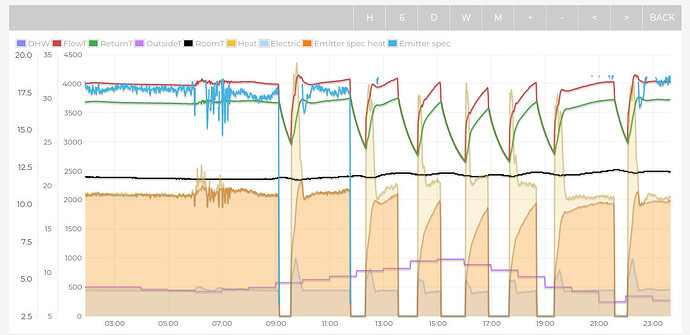
Zoom in to select a part of the cycle where DT is fairly uniform:
During this example period the average flow temperature was 30.3C and return temp 23.9C a mean water temperature of 27.1C. The average heat output according to the heat meter was 4.9KW.
My radiator system cannot output anywhere near 4.9 kW at 27.1C, the actual heat output to the room should be 15.6×((27.1-20)÷50)^1.3 = 1.23 kW. This means that 3.7 kW is going to heating the water, copper and steel in the system.
The 3.7 kW increases the system water temperature by 4.3K over ~10mins
system volume = heat input (Joules) / (4150 J/K.L x 4.3K)
(3700×60×10.1)÷(4150×4.3) = ~125L
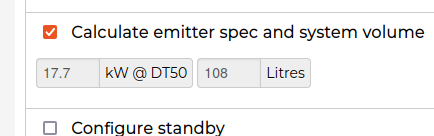
We can use this value to work out the effective system volume, which in this case the calculation suggests is about 125 Litres… Now my own calculation suggests that my system volume should be closer to 73L, not including the volume in the heat pump heat exchanger itself or the heat capacity of the copper pipe and steel radiators.
Given the wide spread of system volume values that come out of this (for mine 100-134L is fairly typical), it’s not super accurate, it’s more of a rough indication.
I was just trying to get reasonable values earlier and was getting numbers all over the shop 
